newer posts
older posts

Pinephone Pro GPS,Camera

2023-04-02

I recently posted about getting Gentoo running on my Pinephone Pro with Pinephone Keyboard. In that post I had gotten this device to pretty much act like a laptop. I had pretty much every piece of software I have on my laptop up and running on my pinephone, under sway using wayland without Xwayland.

Since then I've been working to get GPS, and then the Camera to work. And I now have both working at a beta level at least. Time will tell how stable my efforts will be. Note that the GPS setup is also largely a prerequisite for using the cellphone modem, though I'm not interested in paying for service at this time, so I haven't tried to get that working.

GPS

I'm going to start with the GPS. Specifically my goal was to get offline puremaps to work with GPS input. gpsd has existed for ages, but modern Linux phone OSes largely use geoclue as their input. Geoclue can determine your location using a GPS, but also by looking up wifi access points and cellphone towers in a database, you can use either the database owned by Mozilla or the one owned by Google. Both have privacy implications. You can also *just* use GPS if you like.

Both the pinephone and pinephone pro use an eg5 modem for talking to cell towers. This modem also embeds a GPS unit as well, so we need to get communciation with the eg25 modem working to use the GPS. A lot of documentation I found about the eg25 modem claims that if you don't see the eg25 modem on your USB bus, the modem is faulty. This is false. There are in fact other reasons the mdoem might not appear.

You'll also need a SIM card for the modem. It doesn't need to be recent or active, any SIM card should o it. It's actually possible to get the GPS to turn on, get a lock, and spit out GPS data without the SIM using AT commands, but it's not possible to get geoclue to talk to it, because it'll only talk to the modem if the modem is "enabled", which requires the SIM card. So, my advice is jsut drop a SIM card in and move on.

Camera

As of the time of writing of this post megapixels supports the pinephone camera out of the box, but not the pinephone pro camera. This will probably be rectified soon at which point you can use the "megapixels" app from the bingch overlay. For now though, you'll need to build it yourself.

Megapixels should now be able to take photos. The green tint is only in the viewfinder, the final images will be properly post-processed and should come out with pretty okay colors.

Kernel config

Here's the kernel config I'm using right now. I'm still building some modules I don't need, but as I get things working it's becoming clearer what I can just remove. pinephonepro-kernel-config-2

One last thing. As I mentioned in my previous post, you need to ensure you're not constantly logging to the internal storage or you'll burn it out. I've set up the systemd journal stuff to only log to memory. I removed virtual/logger and told portage it was installed via /etc/portage/packages.provided, so I have no logger. After trying a few other approaches I made /var/log a small tmpfs. /tmp and /var/tmp/portage are also tmpfses for similar reasons. I tried symlinks and some other tricks for /var/log and none quite worked.

Conclusion

That just about wraps it up for the pinephone pro. At this point we have almost all of the hardware capabilities of any of the main phone linux distributions, but working on Gentoo. The one thing we don't have working is the "cell" part, because right now that's not something I particularly want. But we have the eg25 modem working, so we're a good chunk of the way there.

In addition to the cell stuff there are a couple of things that still don't work.

Otherwise though our phone can do everything (within processing power limits) that a laptop can do, AND several things only a phone can do, has a physical keyboard, and fits in a large pants pocket. This is the closest I've ever gotten to a true convergence device. There are plenty of improvements to be made, but for the first time I have a truly portable linux box that's actually *useful*.

Lastly, here's some useful resources

Linux Software I use

2023-03-24

I find that a large percentage of my time "tweaking" linux is spent searching for software that I like. I've slowly built up a toolset that's lightweight and stable, and does the things that I generally need to do - and I thought that *maybe* someone else would find this list useful too. I am sure there are better ways to do some things, but I'm pretty happy with most of how my setup works.

Rather than try and come up with a list of programs I use, Gentoo already has this list sitting around for me in the form of the world file. For non-gentoo folks, the world file is a list of every package I explicitly installed, and doesn't include dependencies. So it's pretty much everything I use. I did drop some stuff from the list that's just boring, like fonts and git.

One reason this list might be interesting to someone is that this configuration is 100% wayland. I'm not running X or XWayland. So if you're looking for a wayland solution to something, this list might be a helpful list. Another reason is that I strongly dislike heavyweight software. I will generally choose the lightest-weight option available. I do still have thunderbird on this list, I'm not saying I always use the most minimal option. Sometimes I just want to look at a darn graphical calendar, but the bias in this list is clear.

Lastly you may notice that my configuration isn't over-engineered, in fact it's very under-engineerd. I have hard-coded paths just written into scripts. This is partly because this really is what I run, not some cleaned up configuration I created for posting. It's also because I don't like over-engineering. As is I can fix this stuff really easily. My notifications aren't working? Oh yeah, that wav file doesn't exist, eh, just find a new one. It's no harder to modify notifications.py than it is to modify the config that calls it. I'm probably erring too far on the side of lazyness, but hey, it works.

I've dropped some of the less interesting entries from the world file, things like git, fonts, etc.

Not every piece of software I use is in the portage package system (or maybe it is but I haven't got searching for the overlay). I should probably write ebuilds for these and be all cool and Gentooy, but I haven't.

Then I run a few things on my server:

But... how do you configure sway you ask? Never fear, here's my sway and waybar configs from my laptop. It's a pretty vanilla config, not much to see here. The notification bits are probably the most interesting

Then a couple of short scripts I'll just post inline. The volume script is interesting because it changes ALL the pulseaudio volumes. This means shortcuts using it that get attached to e.g. the volume buttons on my phone, work when using plug-in headphones, a bluetooth speaker, USB headphones, or the built-in speaker. I've tried a lot of scripts over the years and this works the best so far. It DOES occasionally cause a weird jump in the settings if something else touched a volume, but I prefer everything to just adjust together for simplicity

volume

change=0 device=alsa_output.pci-0000_00_1f.3.analog-stereo cur_vol=$(pactl get-sink-volume ${device} | awk '/front-left:/{gsub("%",""); print $5}') new_vol=$((cur_vol + ${change})) echo $new_vol pactl list sinks | awk '/Name:/{print $2}' | while read SINK; do pactl set-sink-volume $SINK ${new_vol}% done

lcd_brightness

#!/bin/bash v=$(cat /sys/class/backlight/intel_backlight/brightness) expr $v + $1 >> /sys/class/backlight/intel_backlight/brightness

It's possible to get w3m to display images in foot, even over ssh, which is pretty cool. I can run mutt on my server (I have it both places) and view images in an email if I want! This took a lot of poking around the internet, digging through configs, and guessing to figure out - and I've not seen anyone mention it anywhere, so I'm going to add it here. To convince w3m to display images in foot select "img2sixel" for the "inline image display method". For that to work img2sixel needs to be installed, which is part of libsixel in Gentoo. I have w3m-0.5.3_p20230121 built with imlib, gpm, ssl, and unicode use flags. I also have fbcon set, but I'm 95% sure it's not needed. As long as the terminal supports sixel (a format for displaying images in terminal emulator) it'll work. If it's working w3m www.google.com will display the google logo as an image.

PinephonePro with Gentoo

2023-03-22

In this post I'm going to skip the why and jump to the what. I crosscompiled Gentoo for my Pinephone Pro with Pinephone keyboard, and got it working pretty nicely, and I wanted to document some of the process for others.

Obligatory photo of phone in operation

In retrospect the easiest path to get close to what I'm running would be to install mobian and then just use sway instead of phosh etc. One advantage of Gentoo though is the ability to run wayland-only which saeves on resources. An easier way to get Gentoo would be to just download an arm64 stage 3, downlad a mobian image, replace the userland with the stage3 tarball, and you'd be most of the way there. That is not the path I took though. One downside of that approach is that you aren't left with a matching cross-compiler environment for building packages requiring more than the 4GB of ram on the Pinephone Pro. Whether it's worth the extra effort, is up to you.

What is this post's purpose? I'm an experienced software engineer and long-time Linux user and admin. I've run Gentoo in particular before, and recently came back to it. That said, this was my first time building or using a cross-compiler or doing any sort of project like this. My target audience is folks similar to myself. The following is not intended as instructions for beginners. It's an approximate outline that I hope can help others with similar experience avoid a lot of dead ends, and many many hours of googling, or enable someone with a bit less knowledge to pull this off at all.

I'm kind of joking, but so far I haven't tried to get GPS, or the cell modem to work. My priority was to make it useful like a laptop. I have tried to get the camera to work. The client software is called "Megapixels". It installs fine, and I've heard works on the pinephone, but the pinephone pro needs some kernel patches that are not widely used yet. I DO have full convergence working with the kernel config I posted. I can use a USB or bluetooth keyboard, a USB-C HDMI hub, an external HDMI monitor, a USB keyboard, and an ethernet device built indo the USB-C hub, and it all works great. My bluetooth headphones work well, my USB headset works fine. I have the power-button suspend via elogind. My volume buttons work. I can change screen brightness with keyboard shortcuts. If you DO use my config and do anything I don't, you'll probably need to enable those features :).

Honestly, if you manage to truly brick your phone I'm impressed, it's not easy with the pinephone pro... but should you pull it off you're doing this at your own risk, I'm not liable, not my fault, bla bla bla. This just my blog. I don't know what I'm doing.

obligatory photo of pinephone pro doing convergence stuff

Pinephone Tips

At this point hopefully you have gentoo booting on your pinephone. But that's a ways from having everything you want working. Since we've basically set up the pinephone like a laptop you can test things out on a laptop and then apply the changes to your pinephone if that's helpful. But here's some ideas

As I mentioned I'm using wayland only swayWM. Some software I like

I'm hoping to write up another post about my desktop configuration and using sway soon, for less pinephone specific information. But there you go.

This is not the easiest process, but compared to your average "build your own OS for arbitrary device" project I suspect this is downright trivial. If you're good with linux and understand how it all works this actually isn't all that hard. I had to do a lot of reading to e.g. work out what the cflags should be, which bootloader to use, the best way to get a bootable system, etc. Hopefully this set of psuedo-instructions will save you those headaches and make the project only a little more involved than a typical gentoo install.

Lastly, other people blazed this trail already. First the all the folks who patched the Linux kernel, wrote firmware for the pinephone keyboard, etc. And then folks who build Gentoo for it before me. I just followed in there footsteps. https://gitlab.com/bingch/gentoo-overlay/-/blob/master/README.md is the best resource I found https://xnux.eu/howtos/build-pinephone-kernel.html Is where I found megi's sources (though I ended up using the ones from bingch). Megi did a lot of the work of writing patches and collecting disprate patch sets together to get the pinephone to really work well. I know I used a couple of other sources for gentoo-specific pinephone knowledge, but have forgotten what they worry, so appolagies for not citing you, whoever you are.

String Sorts

2019-01-23

You'll recall in my recent post about Fast Sort we learned that Radix Sort is significantly faster at sorting 32 bit integers than normal comparison based sorts, particularly as the array gets large. A result every computer scientist knows theoretically, yet with median-find we saw how theoretical bounds don't always translate in practice.

Well, a friend asked me, what about sorting variable length strings? Does radix sort still beat comparison based sorts? It's a fair question so, I decided to take a shot at it. And here are my results:

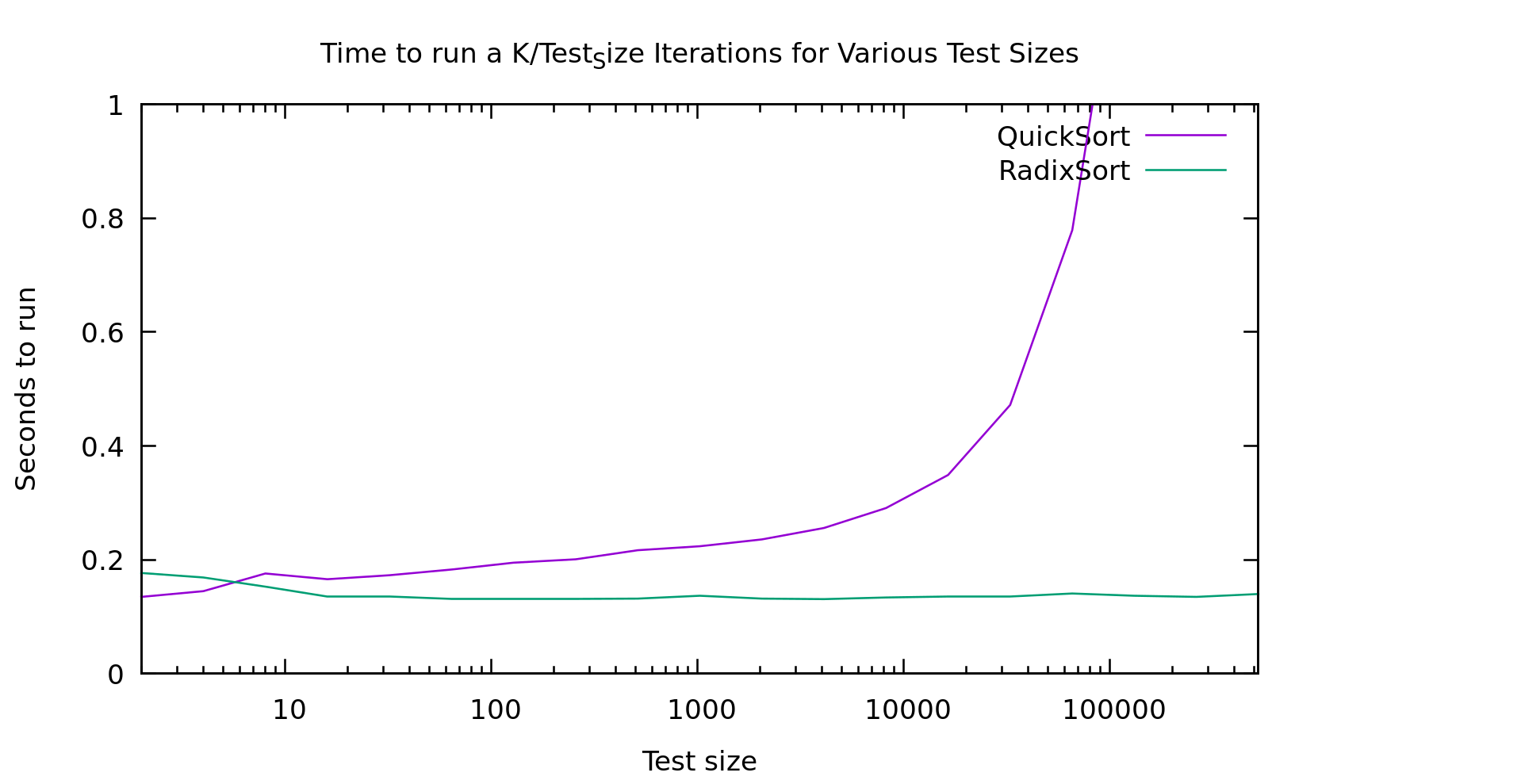
In my mind that graph is pretty clear, above 10 elements radixsort is clearly winning. Note that this is currently a 128 bit radix-sort that only handles ASCII... though I'm actually only feeding it uppercase strings currently. So, lets talk about how this algorithm works, because it's not an entirely trivial conversion of radixsort

String Radix Sort Algorithm

This is a little bit interesting. You may recall that there are two types of radix-sort. Least Significant Digit first, and Most Signicant Digit first. These are referred to as LSD and MSD. My binary radix sort from earlier benchmarks was an example of an MSD sort, and the one I just referred to as "radix sort" is an LSD sort. LSD sorts are preferred generally because they are stable, simplier to implement, require less temp space AND are generally more performant.

There's just one problem. With variable length strings, LSD sorts don't work very well. We'd have to spend a lot of time scanning over the array just looking for the longest array so we can compute what counts as the smallest significant bit. Remember that in lexicographic ordering it slike all the strings are left justified. The left-most charactor in each string is equivelent in precidence, not the rightmost.

MSD sorts, must be recursive in nature. That is, they need to work on only the sublist we sorted in to a certain bucket so far. I'm going to call this sublist a "slice". To keep our temporary space vaguely in order I'm using a total of 5 lists.

Here's the algorithm. Start by looking at the first bytes of the strings. Look in slice list A, and get the next slice. Bucket everything in this slice. Each of these buckets (if non-empty) becomes a new slice, so write strings back out to string list B, and write the index of end each slice in to string list B. Swap lists A and B, move to the next byte, and do it again. We terminate when for each slice it's either of length 1, or we run out of bytes. To see the full implementation take a look at string_sort.h in my github repo .

Conveniently, they way my algorithm works it is in fact stable. We walk the items in order, bin them in order, then put them in the new list still in order. If they are equal there is no point where they'd get swapped.

It's a LOT of temporary space, which is kind of ugly, but it's pretty performant as you saw above. Another optomization I haven't tried is short-circuiting slices of length 1. We should be able to trivially copy these over and skip all the hashing work. Testing would be required to see if the extra conditional was worth it... but It seems likely

Data tested on

To test this I'm generating random strings. It's a simple algorithm where, with a probability of 9/10 I add another random uppercase letter, but always stopping at 50 charactors. I'm mentioning this because obviously the distribution of the data could impact the overall performance of a given algorithm. Note that this means functionally we're only actually using 26 of our 128 buckets. On the other hand, real strings are usually NOT evenly distributed, since languages carry heavy biases towards certain letters. This means my test is not exactly represenative, but I haven't given it a clear advantage either.

Conclusion

I can't say that this is a clear win for Radix Sort for sorting mixed-length strings. The temporary space issue can be non-trivial, and certainly time isn't always worth trading for space. We're using O(3N) additional space for this sort. That said, there are some obvious ways to reduce the space overhead if you need to, e.g. radix-sort smaller chunks of the array, then merge them. Use 32 bit instead of 64 bit pointers, or come up with a cuter radix-sort.

Note that my radix-sort was a mornings work to figure out the algorithm, write and validate an implementation, find a couple optomizations, and benchmark it. I wrote this post later. Oddly "inline" made a huge difference to gcc's runtime (it's needed due to loop unrolling for handling the A/B list cases). In any case, I've little down someone can beat my implementation, and maybe find something using a bit less space. I just wanted to prove it was viable, and more than competitive with comparison based sorts.

Median Find

2018-12-19

Similar to Radix Sort, I thought it might be interesting to see how worst-case linear time medianfind actually performed. Since the algorithm is identical to expected-case linear-time medianfind (usually called quickselect), except for the pivot selection, I elected to add a boolean to the template to switch between them (since it's in the template it'll get compiled out anyway). Before we go in to the results, here's a quick refresher on these algorithms:

Problem Statement

Imagine you have a sorted array. If you want the K'th largest element, you can simply look at the element in location K in the array. Comparison-based sorting an array takes O(Nlog(N)) time (strictly speaking the theoretical limit is log-star, but it doesn't really matter). What if we want to do this without sorting the array first?

Quick Select

Quick select chooses a pivot and runs through the array throwing elements in to 2 buckets... smaller and larger thanthe pivot. Then it looks the number of elements in the buckets to tell which one contains the k'th element, and recurses. We can prove we usually choose a good pivot and this is expected O(N) time. But it's worst-case is much worse.

Worst-case-linear Quick Select

What if we always chose the right pivot? Or at least... a good *enough* pivot. This is how we build our worst-case-linear quick select algorithm. It's a really cool trick, but it's been covered in many places. So if you want to know how it works you can check wikipedia, or this nice explanation .

Real World performance

All of that is great in theory, but what is the *actual* performance of these things... well, in a benchmark, but at least on a real computer.

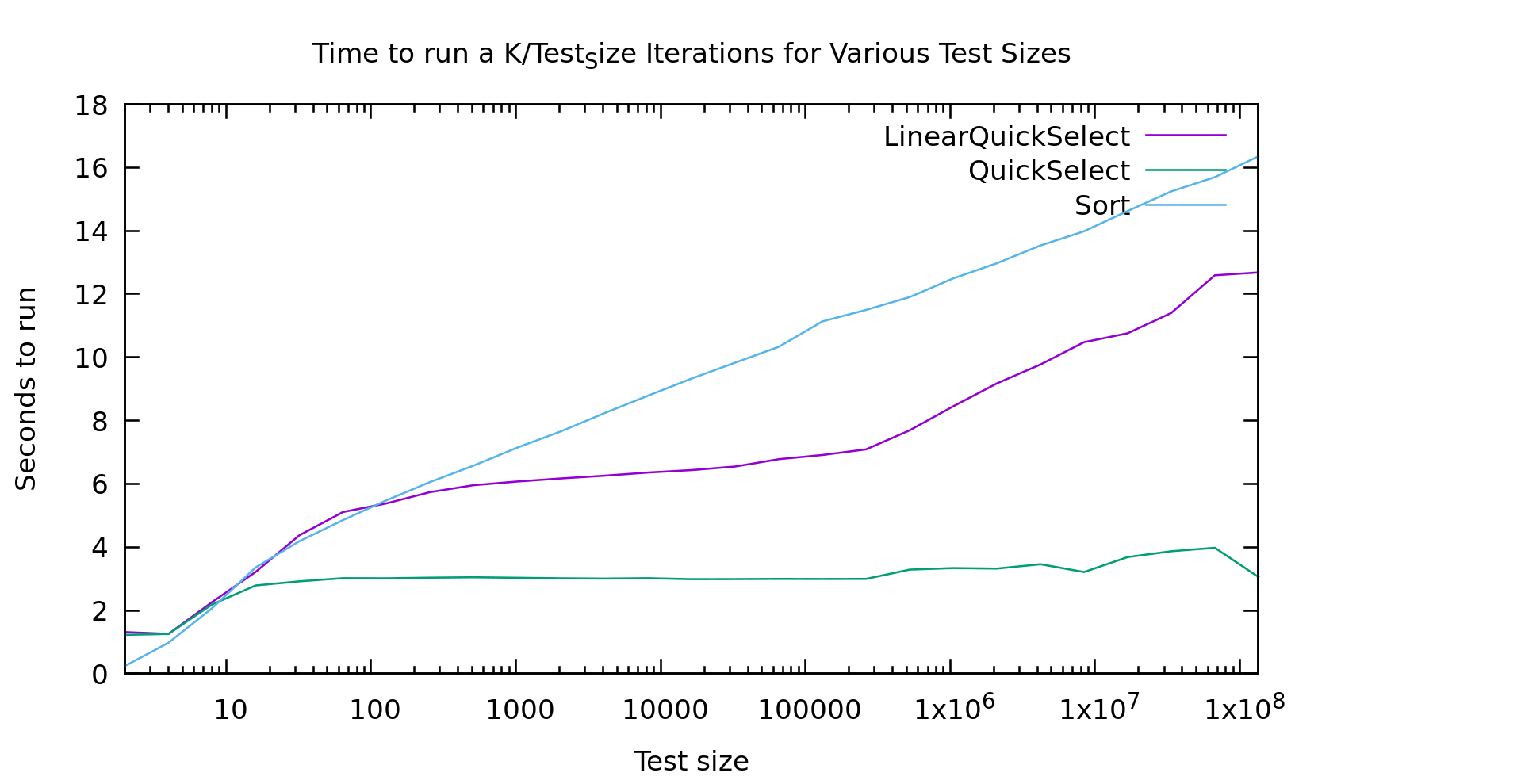
As usual I'm working on an array of test_size k/test_size times, so we work over the same number of array-cells at every point on the graph: small arrays many-times on the left, and large arrays fewer-times on the right.

For a while I was pretty upset about these results. The runtimes for "lineartime" quickselect look more like quicksort (the algorithm displayed as the "sort" line) then they do like basic quickselect. In short... that doesn't look linear at all. What the heck?

I must have a bug in my code right? This algorithm was proved linear by people much smarter than me. So, my first step was to double-check my code and make sure it was working (it wasn't, but the graph above is from after I fixed it). I double, triple, and quadrouple checked it. I wrote an extra unittest for the helper function that finds the pivot, to make sure it was returning a good pivot. Still, as you see above, the graph looked wrong.

I finally mentioned this to some friends and showed them the graph. Someone suggested I count the "operations" to see if they looked linear. I implemented my medianfind algorithm using a seperate index array. That way I could output the index of the k'th element in the *original* array. From there everything is done "in place" in that one index array. As a result, swapping two elements is my most common operation. That seemed like a pretty accurate represention of "operations". So, here's what that graph looks like.

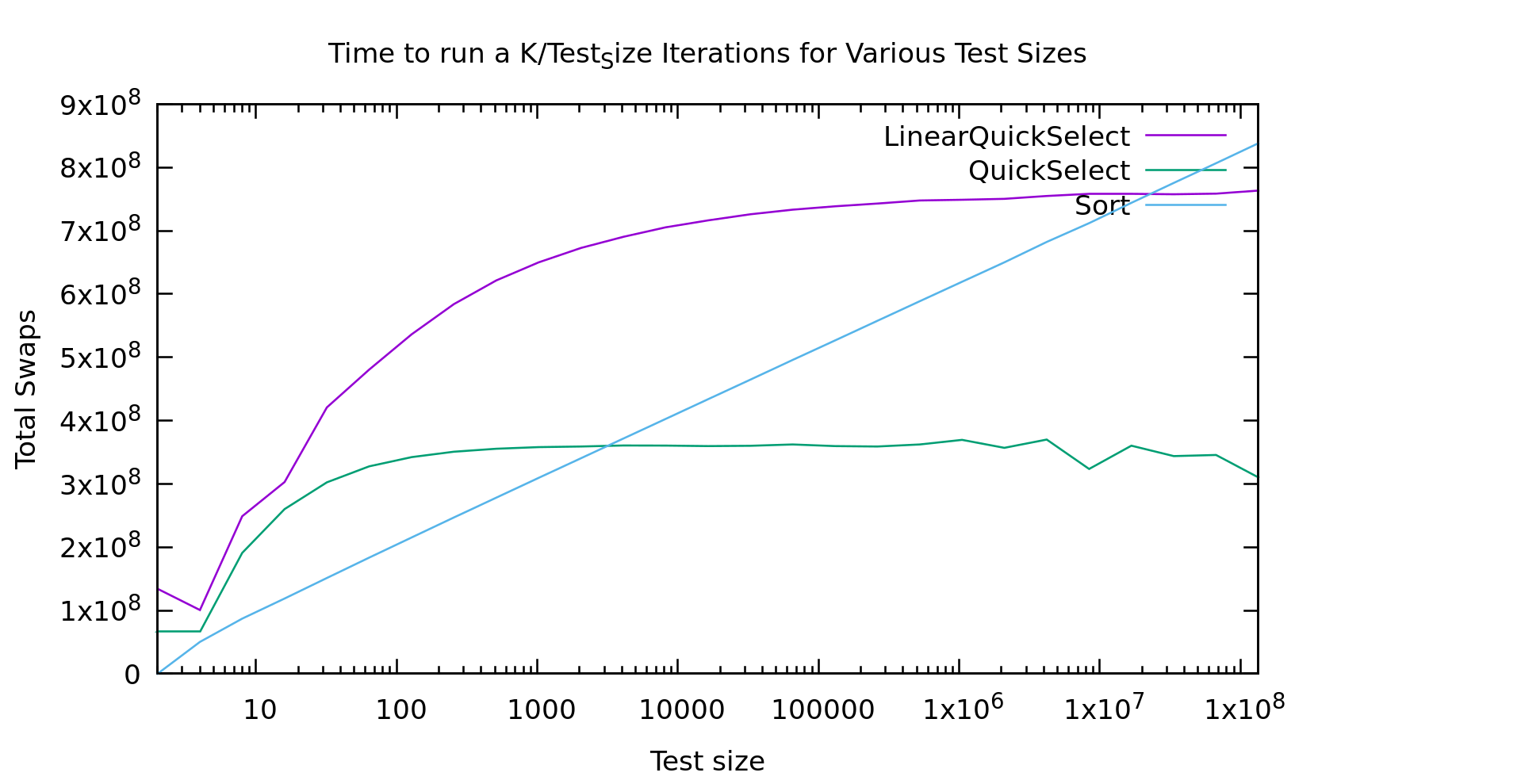
Now THAT look's a bit more linear! It's not exactly a flat line, but it looks asymptotic to a flat line, and thus classified as O(N). Cool... So, why doesn't the first graph look like this?

Real machines are weird. That index array I'm storing is HUGE. In fact, it's twice the size of the original array, because the original is uint32_t's and my index array is size_t's for correctness on really large datasets. The first bump is similar in both graphs, but then a little farther to the right in the time graph we see it go crazy... that is probably the algorithm starting to thrash the cache. Presumably if I made it big enough we'd see it go linear again. That said, if we go that large we're soon running on a NUMA machine, with even more layers of slowness, or hitting swap.

So, should you *ever* use guaranteed linear-time medianfind? Probably not. If there is a case it's vanishingly rare. It happens pivot-selection distributes well, so there's probably a use there? But, if you just used the "fastsort" we talked about in my last post you'd get even better performance, and it's still linear, AND it distributes well too! It's not comparison-based of course, but there are very few things that can't be radixed usefully with enough of them, and if you're stubborn enough about it.

Big O notation didn't end up proving all that useful to us in this case did it? Big O is usually a good indicator of what algorithm will be faster when run on large amounts of data. The problem is, what is large? Computers are finite, and sometimes "large" is so large that computers aren't that big, or their behavior changes at those sizes.

newer posts
older posts